Masonry wall Abaqus simulation

Masonry walls in Abaqus

Masonry can refer to brick, stone, and other building materials. A masonry wall is an arrangement of masonry components such as concrete blocks, burnt clay bricks, sundried bricks, stone bricks, and natural stones joined together with cement or grout. Masonry wall Abaqus simulation is completely presented in the workshops of this package.

Masonry is the oldest structural material that is currently used for a variety of construction projects. It has regularly been applied to a variety of construction projects. Natural stone structures, such as bridges, houses of public and religious worship, and historical monuments, make up the majority of the world’s architectural history. It is crucial to have a solid understanding of the mechanical behavior of stone structures. Their main characteristics are a high compressive strength and a nearly zero tensile strength because of the joints. Therefore, engineers must find strategies to improve these structures’ tensile strength. Look at workshop 3.

As terrorist attacks increase globally, engineers and scientists are paying closer attention to how sensitive infrastructure is to blast loads. Numerous outcomes, ranging from minor damage to structural collapse and substantial human deaths, could result from these loads. Therefore, it is necessary to understand how the structures behave under various loadings. Check out workshops 1 and 4.

You can learn more detail in the description below.

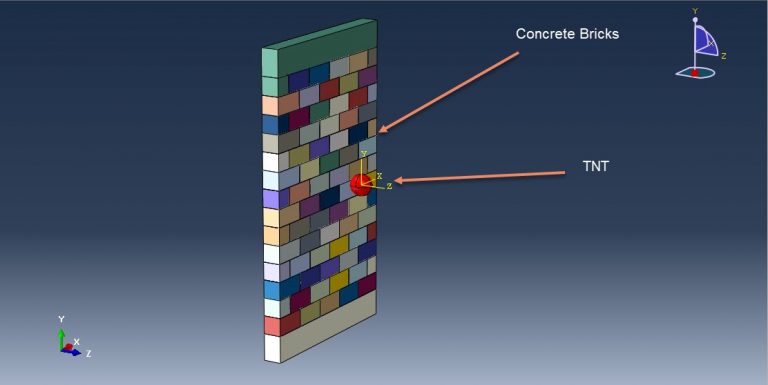
Workshop-1: Masonry wall behavior simulation under coupled Eulerian-Lagrangian explosion

This lesson uses Abaqus to model the behavior of a masonry wall under a couple of Eulerian-Lagrangian explosions. Numerous dynamic issues related to explosions, such as gaseous or condensed charge detonations followed by pressure wave propagation through the surrounding air, are widely solved using numerical models. These techniques can be used to assess the pressure wave’s direction of propagation and the impact it has on various building constructions. In an effort to understand the precise mechanism of the blast phenomenon and develop reliable numerical modelling techniques to predict the response and ultimate failure of the obstacles, researchers have been conducting and publishing numerous real-world experiments along with numerical explosive simulations for various types of materials for many decades. It goes without saying that the type and strength of the charge affect how an explosion will behave in a structure. These two factors can be changed and get quite different outcomes. The purpose of tackling such an issue is explained in the current study, which also provides state of the art in the relevant field.

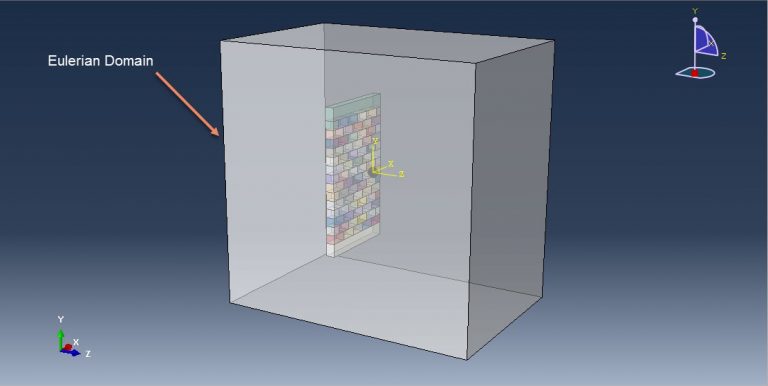
TNT is represented as a solid and the domain as an Eulerian part, while the concrete blocks are modeled as three-dimensional parts. Figures concerning the assembled parts are shown below.

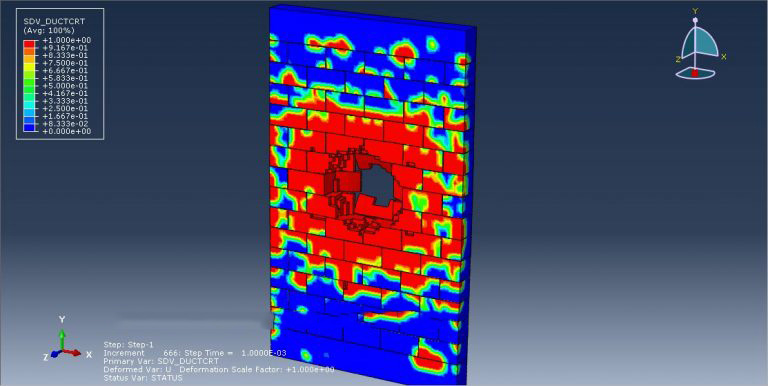
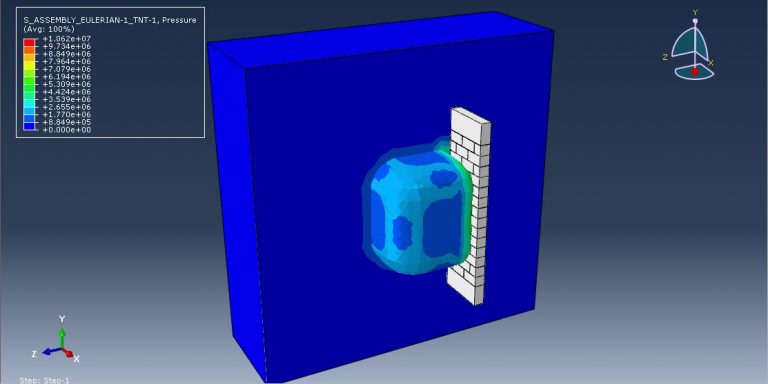
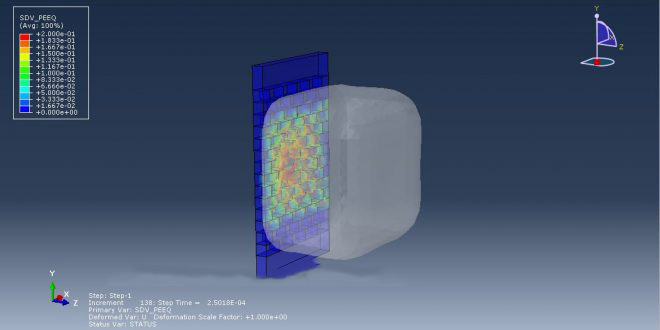
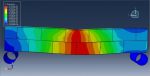
This work’s main goal is to create a solid numerical model of a masonry wall that accurately depicts the behavior of a real building that has been subjected to a CEL explosion. The simulation also heavily depends on the damage value for masonry walls. Using the Johnson-Holmquist model, concrete block behavior at a high strain rate is modeled. It is implied that the JWL equation of state can model TNT behavior. There is the use of a dynamic explicit process with all contacts. The cohesive property as cohesive, damage with evolution, and tangential friction are utilized to characterize mortar behavior in interaction. The contact surfaces of blocks are given this attribute. The bottom concrete beam is subjected to the appropriate boundary condition. The volume fraction approach is used in this tutorial to specify the position and quantity of TNT. The output results are much improved by the fine mesh.

Following the brick simulation, it is possible to achieve pressure, stress, and TNT wave. Below are some results’ figures that you can see.

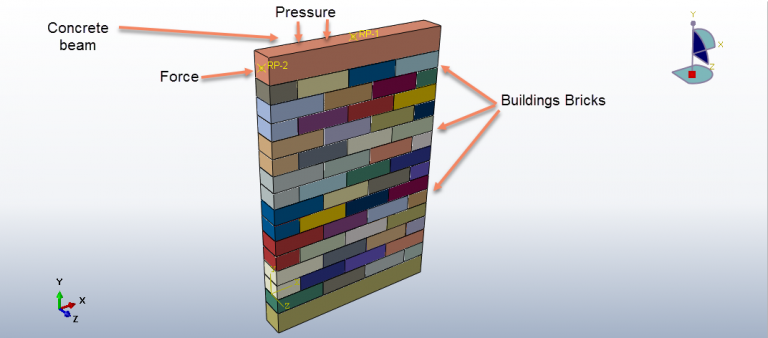
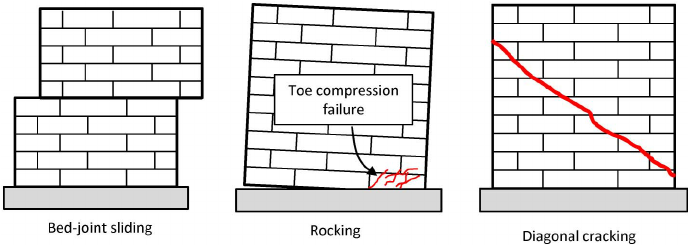
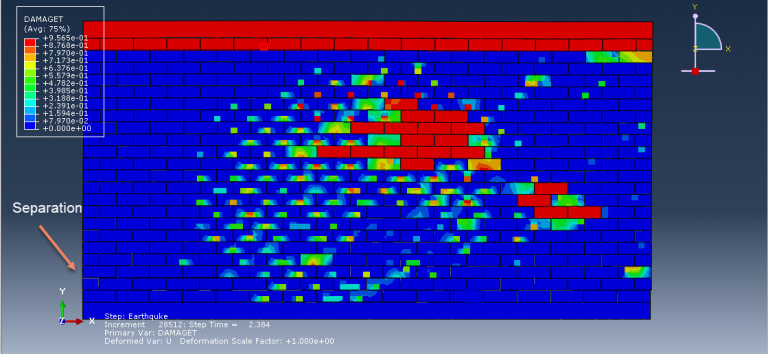
Workshop-2: Micro modeling of masonry wall (brick wall) in Abaqus and investigation of rocking mode fracture

The earliest structural material that is still in use for a wide range of construction is masonry. It has consistently been used for various building tasks. Old masonry structures were designed and built by skilled masons using trial and error techniques rather than complex calculations. Engineers have recently focused their attention on innovative masonry structure analysis techniques. Overall, interface features are needed in a numerical model to better accurately represent masonry’s response. The use of interface components for the joints and suitable models for masonry units, grout, and mortars are two potential options.

Bricks were modeled in three dimensions for this simulation. The position of the bricks and concrete beams is shown in the below diagram. Concrete and bricks are regarded to be elastic materials.

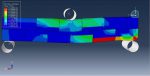
There are two ways to simulate the behavior of mortar. Because mortar underwent damage during analysis, it should be taken into consideration. The first method defines the mortar as three-D parts. The second method assigns it to the bricks’ contact surfaces by coupling cohesive behavior with damage and evolution. The second approach has been utilized in this simulation, and BK Low considers it to be a damage formulation with normal, shear, and tangential stiffness.

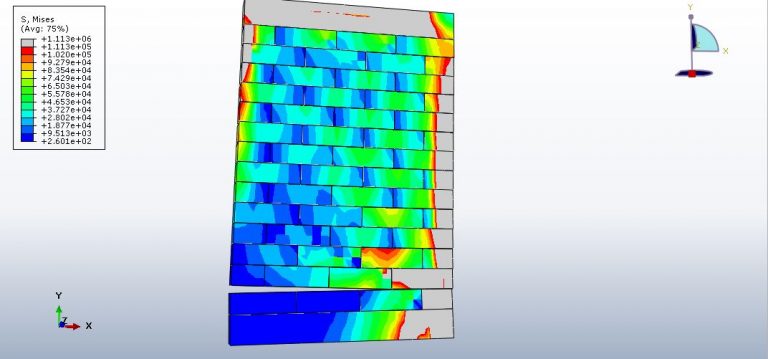
You can see some figures about the outcomes of this simulation below. Rocking failure mode was detected during analysis by applying normal pressure and concentrated transverse force.

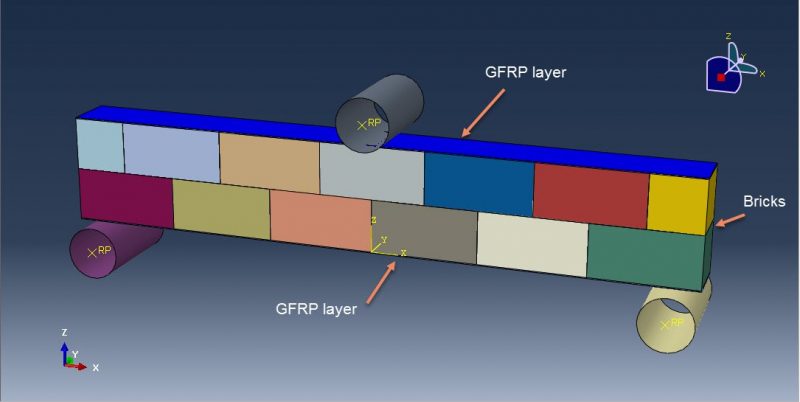
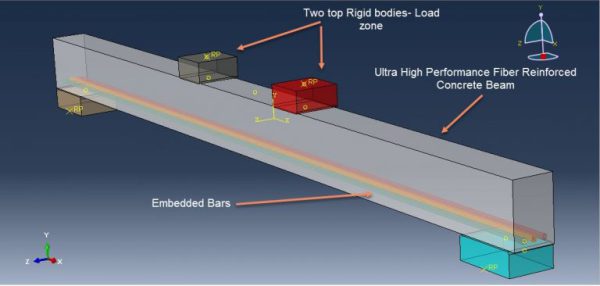
Workshop-3: Numerical simulation of reinforced bricks masonry beams using GFRP reinforcement in Abaqus

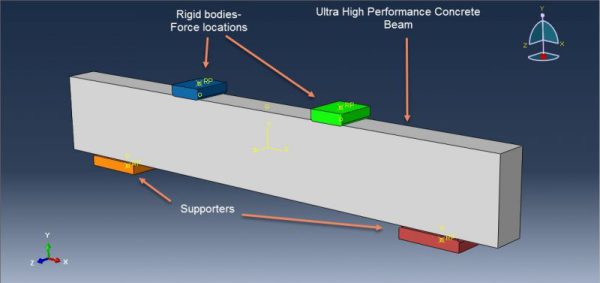
This lesson has looked at the numerical simulation of the masonry wall Abaqus built of reinforced bricks masonry beams using GFRP reinforcement. Both the GFRP sheets and the bricks are modeled as three-dimensional solid components and shell parts, respectively. A diagram of the assembled parts is shown below.

The majority of the world’s architectural history is made up of natural stone constructions, including bridges, places of public and religious worship, and historical monuments. The advantages of natural stone are its beauty, accessibility, hardness, toughness, and sustainability. A thorough knowledge of the mechanical behavior of stone constructions is essential. Due to the joints, their primary traits are high compressive strength and nearly zero tensile strength. Therefore, only members who primarily work in compression are allowed to use stone in historical constructions. One of the techniques most frequently utilized in the restoration of historic buildings with the goal of enhancing resistance is the reinforcing of masonry structures. Steel bars, rings, and/or composite materials may be used in its execution. Composite materials, like FRP, have come into more and more consideration over the past twenty years for strengthening and repairing both new and old masonry structures. Due to their high tensile strength, resistance to corrosion, and ease of handling, FRP make great candidates for strengthening. The strengthening of masonry with composites reinforced with carbon and glass fibers has been covered in a number of studies.

The bricks exhibit elastic behavior with concrete damaged plasticity. The GFRP sheets use the elastic behavior lamina type with Hashin failure criteria. It uses the explicit step with all contacts. It is expected that the contact between the blocks and the GFRP sheet is flawless. Through the use of cohesive behavior and damage parameter, mortar is used as a cohesive surface contact. For the top rigid body to exert force, displacement is used, whereas the fixed boundary condition is used for the bottom rigid bodies. To get good results, the mesh needs to be fine.

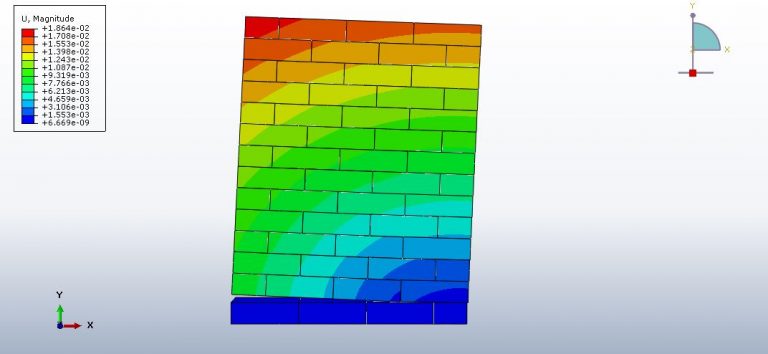
All findings, including stress, strain, displacement, etc., are attainable after the simulation. Below are some figures that represent the outcomes.

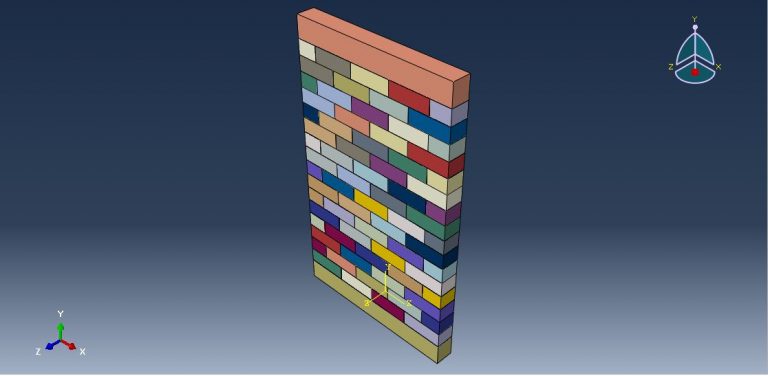
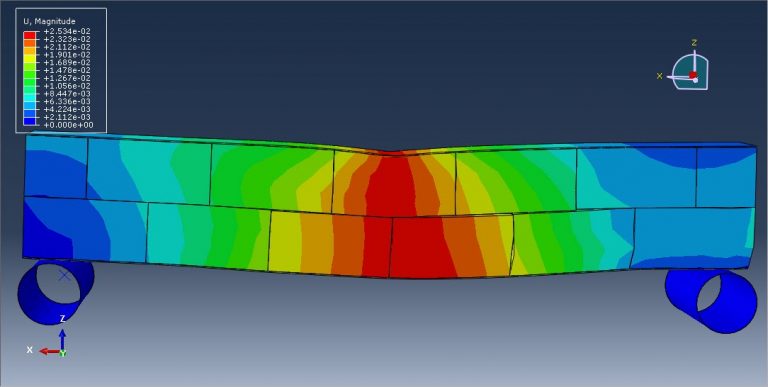
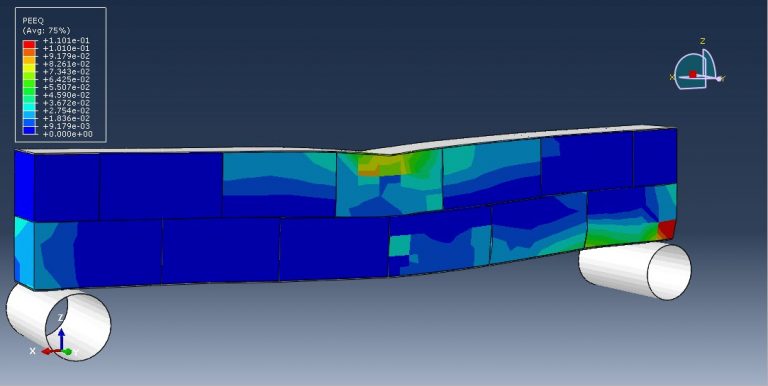
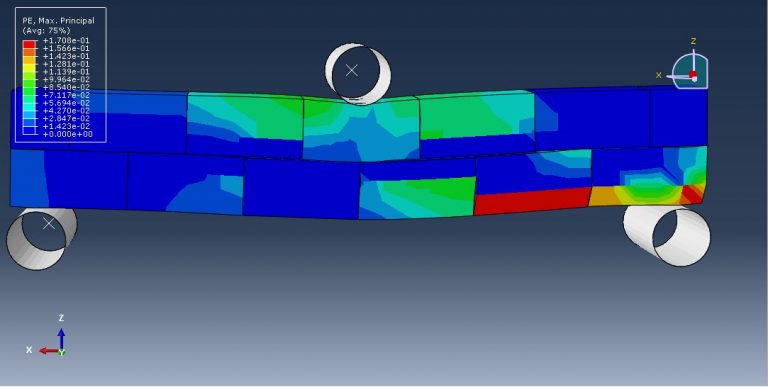
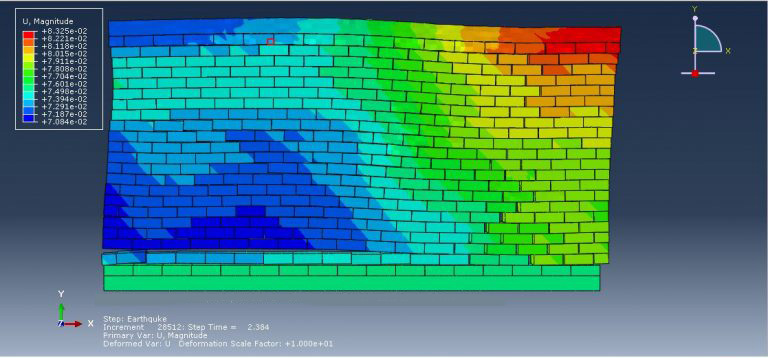
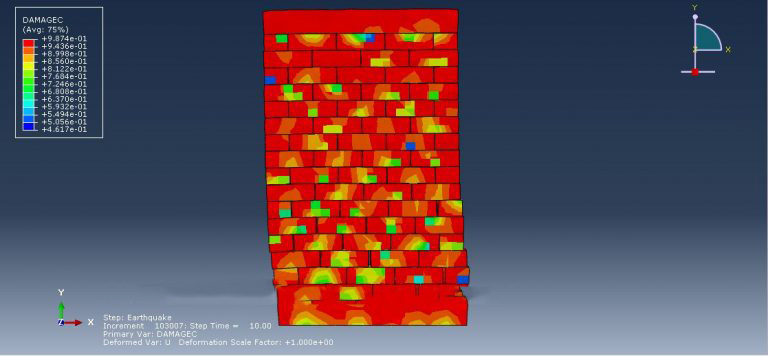
Workshop-4: Abaqus micro model simulation of earthquake load over a masonry wall

Engineers and scientists are paying more attention to how vulnerable buildings and infrastructure are to blast loads as terrorist attacks increase on a global scale. These loads could have a variety of consequences, from minor damage to structural collapse and significant human casualties. The oldest and most popular construction material, masonry, which is utilized in both masonry buildings and infill walls in buildings made of reinforced concrete (RC) frames, sustain the most damage. Even without total destruction or structural collapse, the flying debris may result in serious fatalities or injuries. As a result, various researchers made an attempt to look into workable ways to strengthen masonry walls in order to improve their resistance to blast loads. The application of fiber-reinforced polymers (FRP) to the surface of unreinforced masonry (URM) walls is one of the most often used methods of upgrading URM walls, despite the fact that a number of procedures have been tested. A wall’s flexural resistance needs to be improved since the blast puts pressure on the wall’s surface.

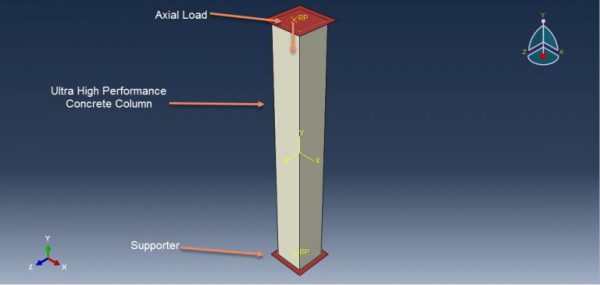
In this simulation, two scenarios were examined: the first involved an earthquake load applied to a masonry wall, and the second involved a pressure and transverse load. The concrete column and bricks are represented as three-dimensional elements in the model. Below is a picture of the finished product.

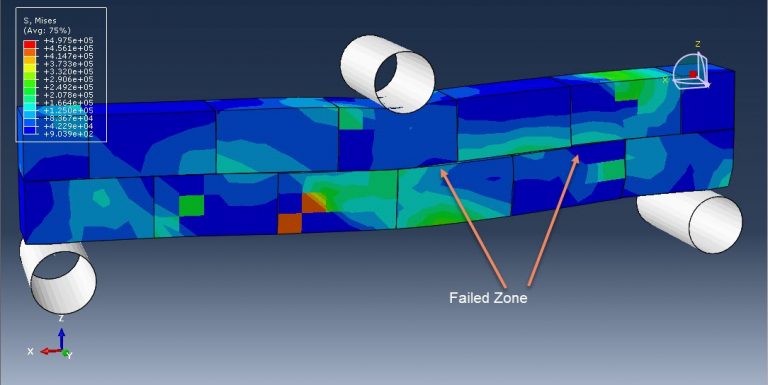
To replicate the first example, three dynamic explicit steps were employed, and one step was used to represent the second phase. There are two ways to model the mortar. The first method involves placing a solid portion between the block’s horizontal and vertical contact surfaces and giving it traction separation behavior. The second method takes into account mortar behavior by using the cohesive contact property, three stiffness data, and damage with evolution. The second option is chosen in this simulation. The gap between the block contact surfaces and the mortar has collapsed as a result of the enormous deformation caused by the seismic stress during the simulation. Below are some results figures that you can see.

It would be useful to see Abaqus Documentation to understand how it would be hard to start an Abaqus simulation without any Abaqus tutorial.