The package aims to teach a specific subject
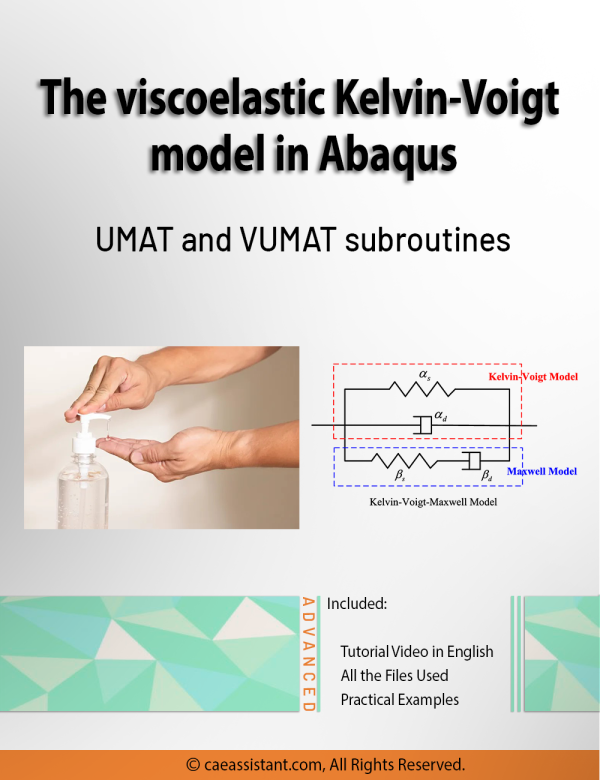
Abaqus Kelvin Voigt Viscoelastic Simulation Using UMAT and VUMAT Subroutines
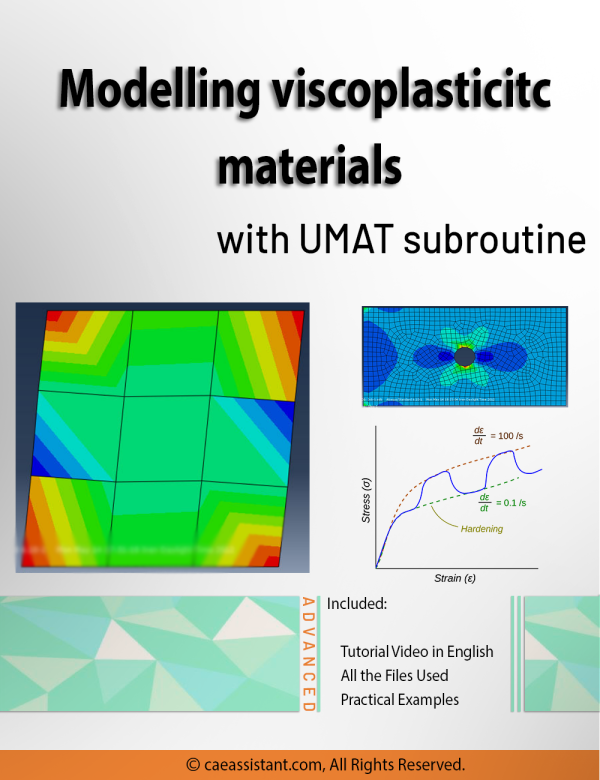
Viscoplasticity Abaqus Simulation Using UMAT Subroutine | Perzyna Viscoplastic Model
Viscoplasticity describes the rate-dependent inelastic behavior of materials, where deformation depends on both stress magnitude and application speed. This concept is crucial in many engineering applications, such as designing structures under dynamic loads, modeling soil behavior during earthquakes, and developing materials with specific mechanical properties. Viscoplasticity Abaqus simulation, especially using Abaqus with UMAT subroutines, are vital for understanding, predicting, and optimizing the behavior of viscoplastic materials. This tutorial focuses on implementing the Perzyna viscoplasticity model in Abaqus. The Perzyna viscoplastic model, a strain rate-dependent viscoplasticity model, relates stress to strain through specific constitutive relations. This involves defining plastic strain rate based on stress state, internal variables, and relaxation time. The tutorial provides general UMAT codes for viscoplastic analysis, yielding results like stress fields essential for various engineering applications. These simulations help in predicting permanent deformations, assessing structural failure points, and analyzing stability under different loads, benefiting fields such as aerospace, automotive, civil engineering, and energy.
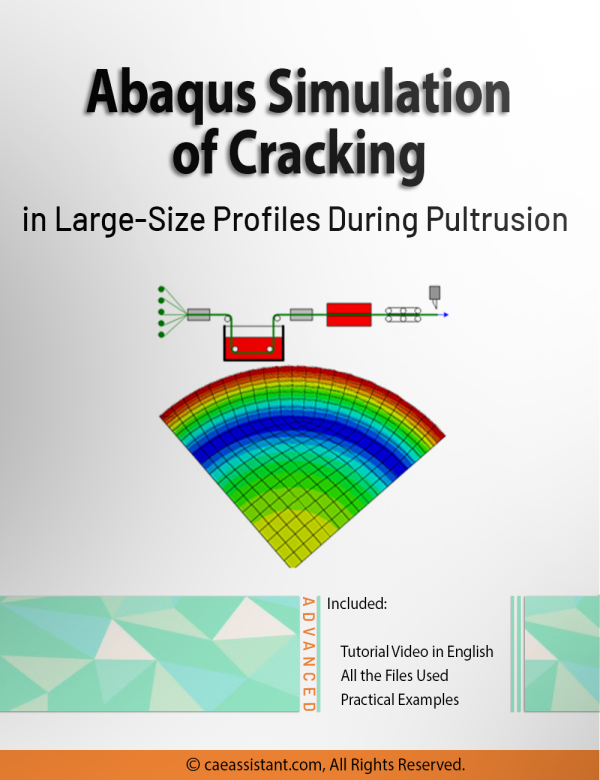
Pultrusion Crack Simulation in Large-Size Profiles | Pultrusion Abaqus
Pultrusion is a crucial task for producing constant-profile composites by pulling fibers through a resin bath and heated die. Simulations play a vital role in optimizing parameters like pulling speed and die temperature to enhance product quality and efficiency. They predict material property changes and aid in process control, reducing reliance on extensive experimental trials. However, simulations face challenges such as accurately modeling complex material behaviors and requiring significant computational resources. These challenges underscore the need for precise simulation methods to improve Pultrusion processes. This study employs ABAQUS with user subroutines for detailed mechanical behavior simulations, including curing kinetics and resin properties. Key findings include insights into crack formation (pultrusion crack simulation), material property changes, and optimization strategies for enhancing manufacturing efficiency and product quality. This research (pultrusion Abaqus) provides practical knowledge for implementing findings in real-world applications, advancing composite material production.
Elastomeric Foam Simulation Using Abaqus Subroutines
Abaqus convergence tutorial | Introduction to Nonlinearity and Convergence in ABAQUS
This package introduces nonlinear problems and convergence issues in Abaqus. Solution convergence in Abaqus refers to the process of refining the numerical solution until it reaches a stable and accurate state. Convergence is of great importance especially when your problem is nonlinear; So, the analyst must know the different sources of nonlinearity and then can decide how to handle the nonlinearity to make solution convergence. Sometimes the linear approximation can be useful, otherwise implementing the different numerical techniques may lead to convergence.
Through this tutorial, different nonlinearity sources are introduced and the difference between linear and nonlinear problems is discussed. With this knowledge, you can decide whether you can use linear approximation for your nonlinear problem or not. Moreover, you will understand the different numerical techniques which are used to solve nonlinear problems such as Newton-Raphson.
All of the theories in this package are implemented in two practical workshops. These workshops include modeling nonlinear behavior in Abaqus and its convergence study and checking different numerical techniques convergence behavior using both as-built material in Abaqus/CAE and UMAT subroutine.
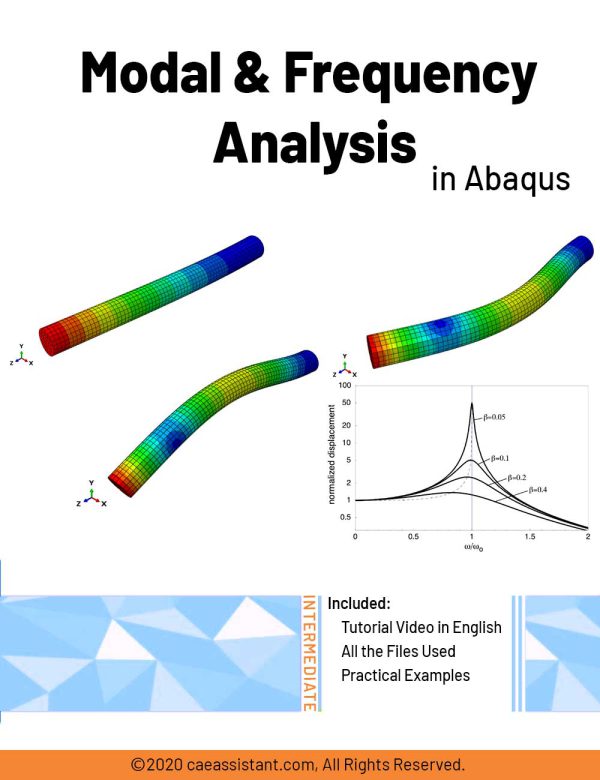
Modal and Frequency Analysis in Abaqus | Abaqus modal Analysis
Simulation and analysis of a 6-cylinder V engine with MSC Adams
Short fiber composite damage (Mean Field Homogenization Model)
Tread wear simulation in Abaqus
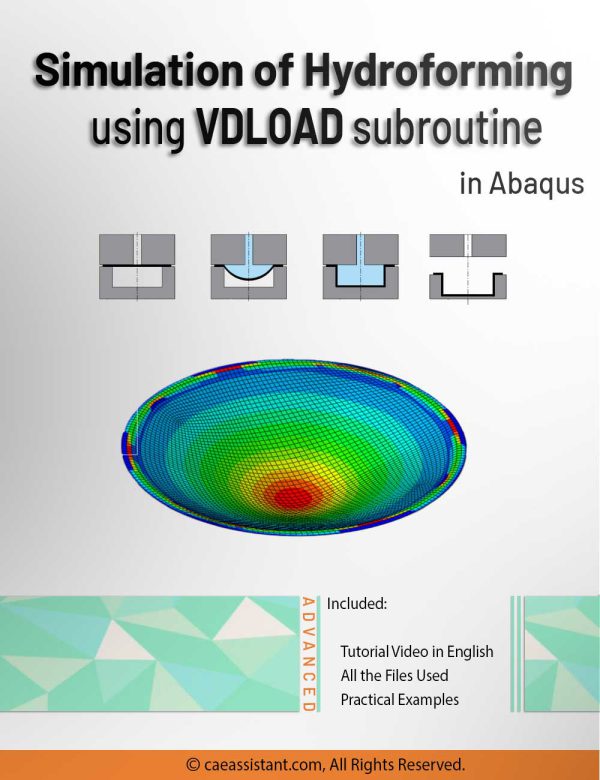
Hydroforming process simulation using VDLOAD subroutine in Abaqus
Topology Optimization in Abaqus
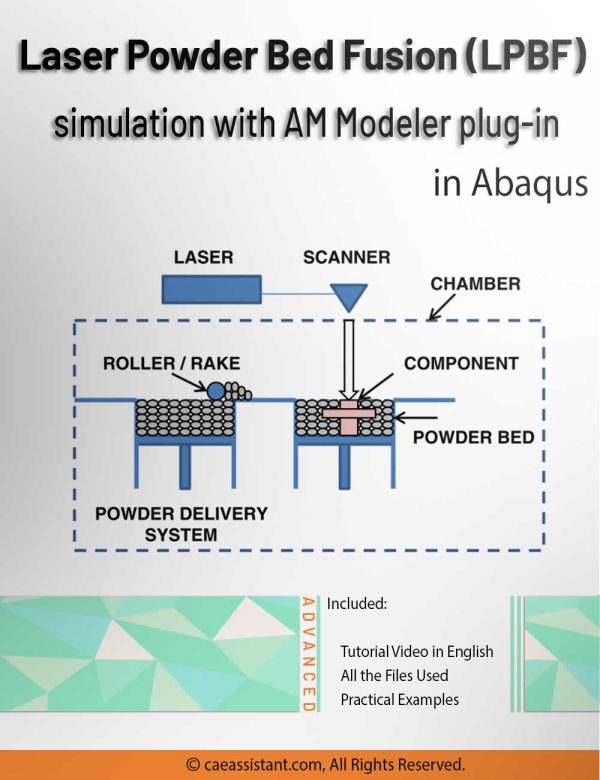
3D printing simulation with Laser Powder Bed Fusion (LPBF) method in Abaqus
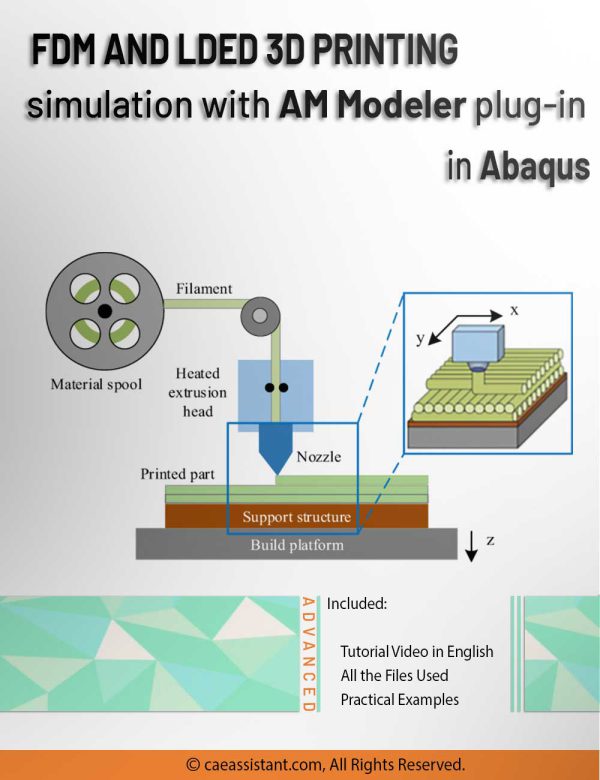
3D printing simulation with Fused Deposition Modeling (FDM) in Abaqus
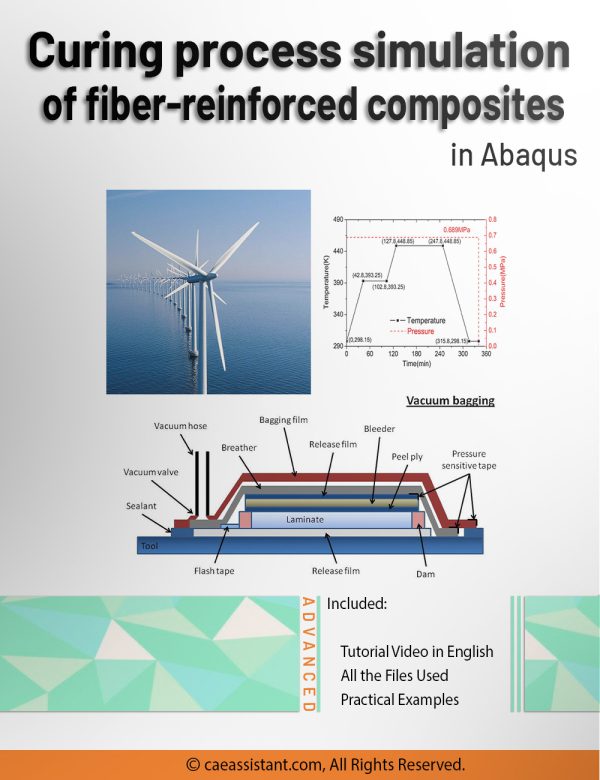
Curing process simulation in Abaqus
Different Techniques for Meshing in Abaqus
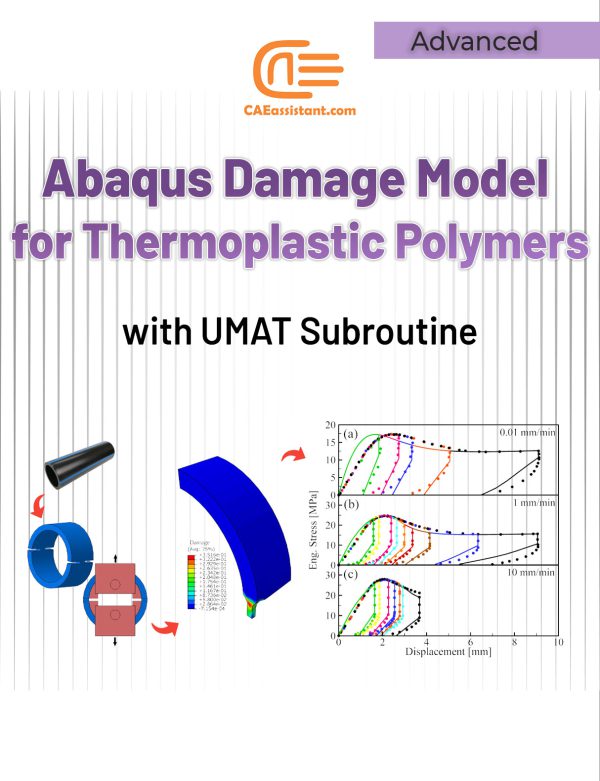
Abaqus Damage Model for Thermoplastic Polymers with UMAT Subroutine
Simulation of shape control by piezoelectric in Abaqus
Techniques of simulating Large and Complex models in Abaqus
Piezoelectric simulation in Abaqus
Abaqus for Civil Engineering Part-1
DISP and VDISP Subroutines in ABAQUS